PhD defence of David Ruiz

7 December 2020

David Ruiz defends his PhD in Numerical Mechanics and Materials on Dec. 7th, 2020.

"Full field modeling of discontinuous dynamic recrystallization in a CPFEM context"

David Ruiz Sarrazola conducted his PhD work under the supervision of Marc Bernacki (MSR team) in the framewwork of the Industrial ANR Chair DIGIMU.

He will defend his thesis work in Numerical Mechanics and Materials in front of this jury:

– Pr. Laurent DELANNAY (Univ. Catholique de Louvain, Louvain-la-Neuve, Belgique)

– Pr. Lukasz MADEJ (AGH Univ. of Science and Tech., Krakow, Pologne)

– Assoc. Prof. Roland LOGÉ (Ecole Polytechnique Fédérale de Lausanne, Lausanne, Suisse)

– Pr. Javier SIGNORELLI (Inst. de Fisica Rosario, Santa Fé, Argentine)

– Privat Dozen Franz ROTERS (Max-Planck-Institut für Eisenforschung, Düsseldorf, Allemagne)

– M.R. Daniel PINO MUÑOZ (MINES ParisTech-CEMEF, Sophia Antipolis)

– C.R. Aurore MONTOUCHET (Framatome Creusot, Le Creusot)

Abstract of his work:

Dynamic recrystallization (DRX) is one of the main metallurgical phenomena responsible for the evolution of the microstructure of metallic materials subjected to hot metal forming processes. Understanding and predicting the subsequent physical mechanisms is of prime importance as the resulting microstructure will be directly responsible of the final in-use material properties. Thus, numerous phenomenological models (JMAK type for example) aiming to describe DRX have been developed in the state of the art. However, because of the complexity of the mechanisms involved in DRX and their interactions, phenomenological or mean field models are not able to fully account for the local evolution of the microstructure and full field approaches are required generally when precise calculations are aimed. Most DRX full field models have limitations in their ability to model high deformation (which limits their applicability for real industrial thermomechanical treatments) and in their description of plastic deformation (which is often grossly simplified).

In this PhD, a new full field discontinuous DRX (DDRX) model is proposed by coupling a crystal plasticity finite element method (CPFEM) with a level-set finite element (LS-FE) framework to describe the grain boundary network motion. The proposed model considers anisotropic plastic deformation and its impact on grain boundary motion. Combined with a remeshing methodology, the proposed numerical framework is capable of describing DDRX up to very large deformation levels. The model is calibrated and compared against experimental measurements of 304L steel. Moreover, the interest of this strategy (ratio precision/numerical cost) is also discussed comparatively to a simpler approach (CP Taylor approximation). All these developments are realized in a generic CPFEM module easily usable in any FE code.

Keywords: Dynamic recrystallization, Full field model, Crystal plasticity, Level-set, Finite element method, 304L Steel.

PhD Defence of Sebastian Florez

30 November 2020

Sebastian Florez defends his PhD in Numerical Mechanics and Materials on Nov. 30th, 2020.

"Towards highly efficient massive-multidomain simulations in the context of microstructural evolutions"

Sebastian Florez conducted his PhD work in MSR team, under the supervision of Marc Bernacki. He defends his work in front of the following jury:

– Pr. Julien BRUCHON (Ecole Nat. Sup. des Mines de St Etienne-Centre Sciences des Mat. et des Struc./Dept Méca. et Procédés d'Elaboration, St Etienne)

– Pr.O. Jean-François REMACLE (Ecole Polytechnique de Louvain, Louvain-la-Neuve, Belgique)

– M.A. Modesar SHAKOOR (Inst. Mines-Telecom, Lille Douai, Douai)

– C.R. Hugues DIGONNET (Inst. de calcul Intensif-Ecole Centrale de Nantes, Nantes)

– Assoc. Prof.Luis BARRALES-MORA (George W. Woodruff School of Mech.Eng. Georgia Tech Lorraine, Metz)

– I.R. Joëlle DEMURGER (ASCOMETAL France Holding SAS-CREAS, Hagondange)

– I.R. Pascal DE MICHELI (Transvalor SA, Biot)

– I.R. Thomas TOULORGE (CENAERO, Gosselies, Belgique)

Abstract of his work:

Strategic industries make extensive use of metallic materials. Today, there is a strong demand from these industries to predict, during hot metal forming processes, the microstructural evolutions of these materials, which are of prime importance concerning their final in-use properties.

In this context of massive multi-domain problems, numerous full-field approaches that describe grain boundary (GB) network motion at the mesoscopic scale have been developed for forty years. When very large deformations are investigated, as in the context of realistic industrial thermomechanical treatments, the level-set (LS) approach in the context of finite element (FE) formulations and meshing/remeshing algorithms remains the most powerful and versatile numerical tool.

Even if recent improvements were realized (context of DIGIMU software), the main weakness of this approach remains its numerical cost, which limits the number of grains considered (small representative volume elements) and still implies long calculation times, especially in 3D.

In these works, the performance of FE-LS models is studied and a new method denominated ToRealMotion, capable to perform 2D massive multi-domain simulations is introduced. This new method, belonging to the family of front-tracking methods, includes various innovations and has been parallelized. Geometrical properties used in the kinetics are only computed at the interfaces, and GBs migration is defined thanks to a Lagrangian scheme, keeping a FE discretization of the bulk of the grains through the concept of body-fitted unstructured FE meshes. This aspect allows for higher adaptability than traditional Front-Tracking models. Of course, one of the main ambitions of this new approach is the improvement of the computational performance when simulating evolving microstructures, while keeping the precision and versatility of the FE-LS approach. As such, 2D numerical cases in the context of grain growth (GG) and recrystallization (ReX) are provided to prove the efficiency of this new approach. These results show impressive reductions in the computational costs and offer promising perspectives on the modeling of massive multi-domain simulations in terms of numerical performance and precision in the modeling of numerous solid-state phenomena.

Keywords: Finite Elements, Lagrangian Models, Body-Fitted Mesh, Level-set, Multidomain simulations.

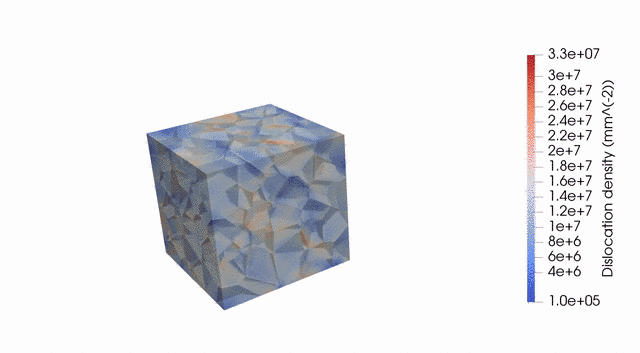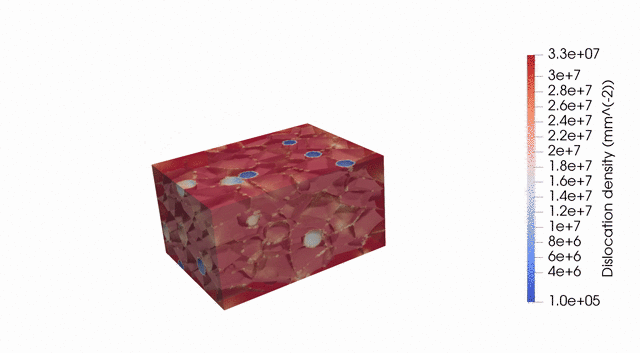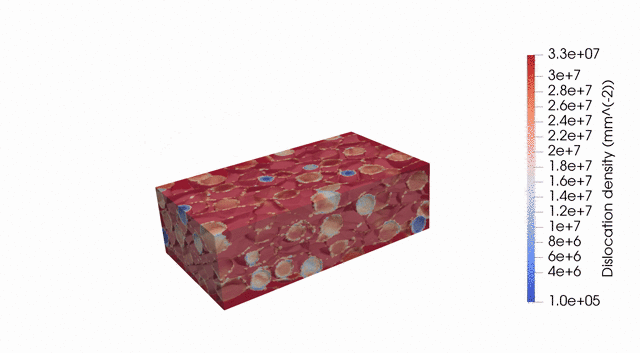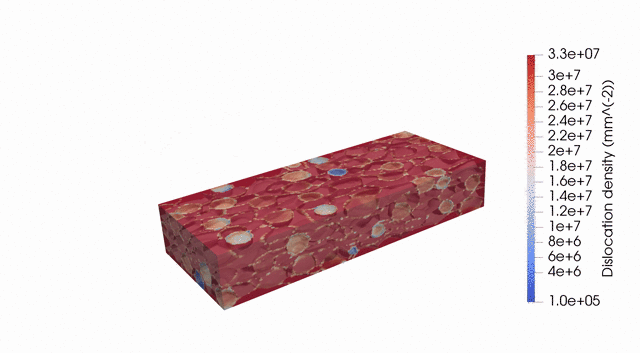
PhD Defence of Shitij Arora

16 November 2020

Shitij Arora defends his PhD in Numerical Mechanics and Marerials on Nov. 24th, 2020.

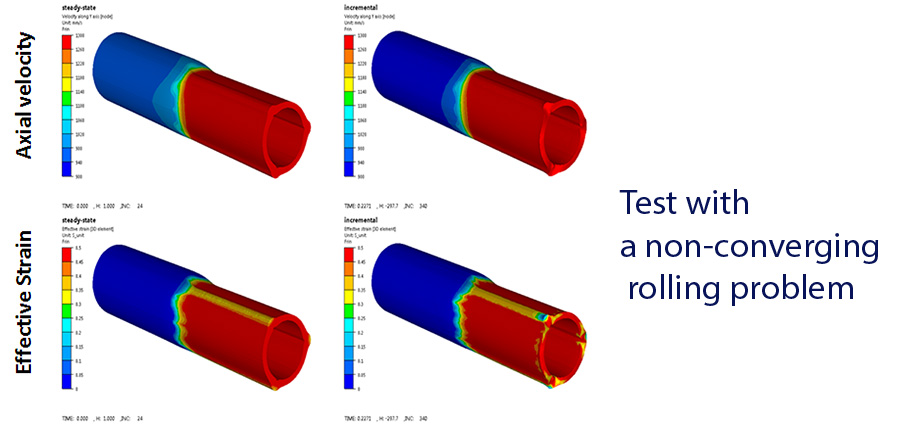
His PhD : Steady-state formulation of metal forming processes: Contact coupling and treatment of history-dependent material models with unstructured meshes

Shitij Arora conducted his thesis under the supervision of Lionel Fourment, who died after a long illness in November 2019. He completed it under the supervision of Pierre Montmitonnet.

He defends his PhD in Numerical Mechanics and Materials on November 24, 2020 in front of a jury composed of :

– D.R. Pierre MONTMITONNET, PhD Director

– Prof. Bert GEIJSELAERS Bert (Twente Univ. Drienerlolaan, Enschede, The Netherlands)

– Prof. Emmanuelle ROUHAUD (Troyes Univ. of Tech., Troyes)

– Prof. Jean-Philipe PONTHOT (Liege University, Liege, Belgium)

– M.R. Katia MOCELLIN (MINES ParisTech-CEMEF, Sophia Antipolis)

– Ing. Ugo RIPERT (Transvalor, Biot)

– Ing. Antoine COURTOUX (Arcelor Mittal)

Abstract:

The existing Fg3stat formulation was proposed for the resolution of the steady-state hot forming processes with viscoplastic materials. The formulation comprises of a strongly-coupled multi-field problem and solved with a fixed-point iterative method. There are two main steps in the iterative algorithm: (i) a thermo-mechanical solver firstly computes the unknown velocity/pressure/Temperature field on a control volume (ii) a free-surface solver then computes the unknown steady-state shape. The Fg3stat, with unstructured meshes based on tetrahedral elements and parallelization with domain partitioning, is found to be at least 50 times faster than the traditional incremental approach for solving these processes. However, the formulation is not robust enough to find a stable solution especially with complex geometries. The work presented in this manuscript is focused on the improvement of the steady-state formulation with two main objectives. Firstly, a consistent contact-coupling is anticipated for a stable solution, and is achieved with a nodal condensation approach. The Explicit and Implicit consistent contact conditions are derived and the updated contact-coupling is tested with complex industrial hot-rolling test cases. Secondly, the original Fg3stat formulation was mainly focused only on hot forming problems with viscoplastic material models which do not consider elasticity effects. These effects become prominent in cold conditions and cannot be ignored. A pseudo-time-step approach makes possible to model the time in the time-independent formulation and is easily adaptable to the existing Fg3stat iterative algorithm. Though originally this approach used structured meshes for tracking streamlines, a new framework is developed to trace the streamlines in the unstructured meshes and to integrate the state-variables for solving the history-dependent problem. In each iteration, the state-variables must be transported from the streamlines to the mesh and vice-versa, which is generally diffusive. To restrict the diffusion, tools like SPR method for field smoothening and P1+ interpolation are invoked. The updated Fg3stat algorithm is tested with different cold rolling problems. The results from the hot and cold rolling simulations with the proposed algorithm are validated with the incremental (time-dependent) solution of the same problem in Fg3inc.

Keywords: steady-state modeling, contact formulation, multi-field formulation, cold rolling

Loss of our colleague and friend, Lionel Fourment

2 December 2019

It is with a great sadness that we learned of the passing on November 30th of our dear friend and colleague Lionel Fourment.

In these painful circumstances, our thoughts are with his wife and his children as well as his loved ones.

As for us, we not only lost a great scientist but also a gentleman full of kindness, caring an optimism about mankind. Tjose of us who were lucky to be acquained with himm will remember a bright mind. We will miss him greatly.

Lionel was a long-time colleague. He had been in the lab. for over thirty years.He joined CEMEF in 1987 and defended in 1992 his PhD work – prepared under the supervision of Jean-loup Chenot – on the topic of "Error estimators and adaptative meshing for computational modelling of forming processes". He spent his entire research career in our lab. He joined CNRS (French National Research Centre for Scientific Research) in 1994 and focused his research activities on the development of numerical methods in solid mechanics: Optimization and identification methods, error estimators, adaptative meshing, solving methods for large linear systems, computation time reduction, parallel computation, contact problems and more recently development of numerical methods for ALE formulation, stationary problems in elastoplasticity. He carried out in-depth investigation on these topics. And we can clearly say that he was one of the key players in the numerical methods developments on our lab. His contributions to the outreach and visibility of CEMEF's scientific production have gone far beyond our laboratory.

He was committed to serving the causes of Science with great humility. He worked for and with the research community of the computational mechanics field, in France and abroad. Being very active in setting up and managing European projects, he became involved with the same enthousiasm in the European ESAFORM association, for which he chaired the scientific awards panels.

We wish to honor his memory. It is still too early to define how this will take place, but we shall keep on promoting and pursuing his work… by organizing among others a scientific seminar in tribute to him, if possible in 2020.

PhD Defence of Luiz Pereira

30 October 2020

Luiz Pereira defends his PhD in Numerical Mechanics and Marerials on Nov. 12th, 2020.

He worked on the mechanisms of oxygen bubble formation in a glass melt in the nuclear waste vitrification context.

His thesis was supervised by Franck Pigeonneau, CFL team in the framework of a project with CEA.

Abstract of his work:

The objective of this project is to determine quantitative parameters inducing the bubble nucleation (gas solubility, supersaturation and nucleation). The impact of the molten glass composition (redox) and temperature will be deeply investigated. Moreover, the role of heterogeneities present in the melt and which may promote nucleation phenomena will be considered. A specific experimental set-up will be implemented in order to in-situ monitor the bubbles formation associated with a molten glass redox state measurement. Post-mortem analyzes on the solidified material will also be performed (electronic microscopy, gas analyzes). In a second step, a basic modelling of the bubble nucleation may be considered using the previously acquired experimental results.

Keywords:

Oxide melt
Bubbles
Redox reaction
Experimental approach
Numerical approach

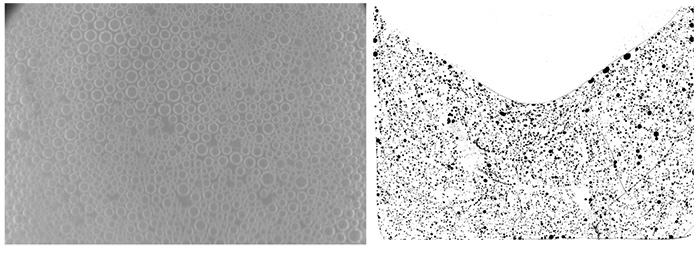
On the left, a population of bubbles in a borosilicate melt at 1200°c, in situ camera imaging device
On the right,a population of bubbles in a borosilicate melt at 1050°c, optical microscopy

Elie Hachem, distinguished 2020 IACM Fellow

7 August 2020

Elie Hachem, scientist at CEMEF, head of the CFL team, is distinguished IACM 2020 Fellow.

The IACM, the International Association for Computational Mechanics, is THE scientific society in the field of computational mechanics. It brings together more than 5,000 members worldwide. Every two years, it awards its prestigious Fellow Award.

This award recognizes up to 8 individuals who have distinguished themselves by their research achievements and publications in the fields of computational mechanics, and who have supported the IACM as members and by taking active part in its meetings and activities.

Elie Hachem talks about this award :

To be recognized by one's peers is always an immense satisfaction. But it goes beyond that. I am looking forward to joining this club that I have always admired. The IACM is THE international community for mechanics and numerical simulation. When I look at the list of winners, among them are my mentors. I think of C. Farhat and J.L. Chenot (former director of CEMEF) to name but a few, but there are many other researchers I admire. This means a lot to me.

I take this award as recognition of the work done, of course. It also strengthens France's position in this field of research. I am proud to contribute to it. It makes me even more motivated to continue to challenge myself and my team on a scientific level.

This prize also gives me a great responsibility. I hope to be able to inspire young people, to make them want to docomputational research.

>> Read more:

– Elie Hachem's page

– Elie Hachem's latest publications

– IACM Fellow Award