PhD Defence of Thibaut Devos

Flux-corrected transport strategies for continuous finite element approximations of the compressible Euler equations on unstructured meshes

Thibaut Devos conducted his PhD work in the CFL team under the supervision of Elie Hachem and Aurélien Larcher. He will defend his PhD in Computational Mathematics, High Performance Computing and Data, on December 4, 2024 in front of the following jury:

Abstract:

This thesis aims to establish a digital framework for addressing industrial issues related to compressible flow. These flows, characterized by significant variations in density and pressure, pose challenges for modeling and numerical simulation due to the formation of shocks and other discontinuities. In this framework, it becomes imperative to develop new digital tools capable of accurately capturing these complex phenomena. This initiative responds to the need to develop new numerical solvers to enrich the range of methods currently employed within our team. These solvers will be designed to solve both scalar conservation laws and inviscid Euler equations, which describe the behaviors of compressible fluids.
The flux correction method for finite elements emerges as a promising approach to overcome these challenges. By first focusing on satisfying physical properties through numerical solutions, notably the conservation of physical quantities such as mass, energy, and entropy, this method ensures a coherent representation of physical phenomena. Once this step is accomplished, it then seeks to optimize the accuracy of results by minimizing numerical errors.

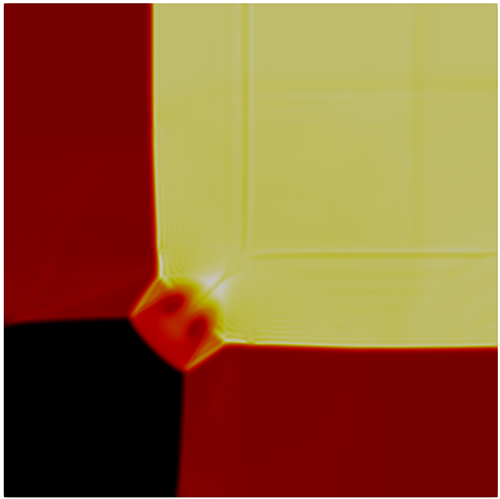
Solution of a 2D Riemann problem for compressible Euler equations

Keywords: Fluid Mechanics, High Performance Computing, Flux Correction, Compressible Flows, Entropic Viscosity